St. Mary's Chapel grid - 3 by 1 diagonal

In this post I shall describe the first two elements of the 'Reshel' basic grid, which is the work of William S. Buehler, previously mentioned, constructed by me on the 3 by 1 diagonal system shown in the previous post.

The first element is the Great Pyramid triangle from the two base points, Kelso and Ericstane:



This point proves to be Hillend Fort(HF), a very significant point in the landscape mentioned previously.

The simplest way to calculate this point is to use trig. function tangent of the Great Pyramid angle, 51d 51m 51s, or 51.86416667 degrees, multiplied by the distance Ericstane(E) to base centre(C), to obtain the point on the axis through St. Mary's Chapel(SM):

For this exercise I have used the St. Mary's Chapel(SM) - North Berwick Law(NBL) orientation which gives a 3 by 1 diagonal to O.S grid of 17.4742 degrees, for now, as it is between the other two options, the Bass Rock, and the Roseline through Arthur's Seat.

The distance from E - C is [{16*sq.rt.3}/2]*(sq.rt.10)/2, which is 21.909miles(E), or 352.59 O.S units, (hundred metres)

tan 51.86416667 * 352.59 = 449.10 O.S.units.

The x and y components can be calculated using the 17.4742 deg. offset angle.

x: sin 17.4742 * 449.10 = 134.85 O.S.grid units

y: cos 17.4742 * 449.10 = 428.38 O.S.grid units.

The calculated base mid - point(C) at this orientation was calculated as 3380.88 6233.87; approximately NT 381 234.

Due to the north west north slant the x component will be subtracted, the y component added:

x: 3380.88 - 134.85 = 3246.03

y: 6233.87 + 428.38 = 6662.25

I have Hillend Fort(NT 245 662) as 3245.50 6662.25 universal O.S. grid coordinates, a mere 50 metres west on the x-axis, and exact on the y-axis. The Roseline orientation would take this towards the west a tad. I will get back to this later, to check!

This second graphic shows two grid connections for this point to within fractions of a degree, which could be used for any practical purpose, for this awkward point.

The line from KA(Kelso approx.)through Hillend coincides with the 2 by 3 diagonal to Blackness Castle, and as can be seen the 2 by 1 diagonal connects at the same point.



I have just noticed the 2 by 1 connection, which only reinforces my opinion of the importance of Hillend Fort, and even more convinced of the practicality of using the 3 by 1 grid diagonal, and indeed this particular grid. Found on Bornholm, applied to Scotland, and defining a specific, and very special point in the construct provided by William Buehler. Wow!


This third drawing is simple in comparison. It is the point which forms an equilateral triangle with the two base points.



Again using the half base length, 352.59 O.S units and the tangent of 60 degrees, defines the point on the axis:

Tan 60 * 352.59 = 610.70 O.S. units
x and y components found using the offset angle of 17.4742 degrees:

x: sin 17.4742 * 610.70 = 183.38 O.S. units

y: cos 17.4742 * 610.70 = 582.52 O.S. units

and again using base centre:

x: 3380.88 - 183.38 = 3197.50

y: 6233.87 + 582.52 = 6816.39

Oxcars (NT 202 817) a tiny island in the Forth is the nearest land, about 0.5 km, but the line clips Inchcolm at the eastern end, a distance of approx 0.75 km.

St.Mary's Chapel grid, continued!

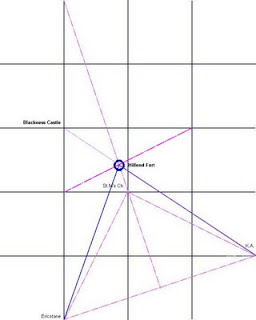
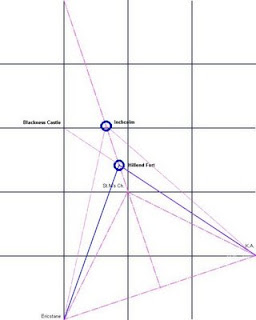
The set of graphics below show first what has been established previously, the first two, and then the extension of the inner smaller derived grid, in (1/sq.root two) relationship, using Blackness Castle, and the simple use of grid diagonals, (2 by 1) and (3 by 1) to find a remarkable system linked to the work of William S. Buehler, whose original work, the ''20 mile radius'' system centred on St. Mary's Chapel. The square on this circle, with The Bass Rock as north-east corner, and the subsidiary system oriented on North Berwick Law, and indeed another with Arther's' Seat summit as main North axis at the half-radius point, (which incidentally has Rosslyn Chapel on it, discussed previously) with a spread of approximately 2.5 degrees is shown in the first sketch.

Sketch One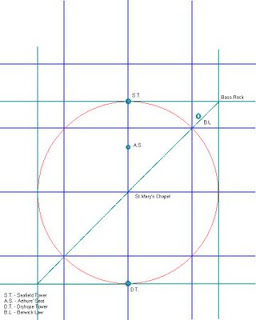



This shows the original circle and square grid derived from it, with a few points marked, St. Marys Chapel(NT275 570) in the centre, The Bass Rock(NT602 873) at the north-east corner, with North Berwick Law(NT556 842) indicated, Seafield(NT279 885) and Dryhope Towers(NT267 247) marking the north and south points respectively. The Arther's' Seat area is marked, and is at the half-radius point, but not indicated as such, but may be considered further, but later! The derived inner grid is found naturally in the geometry!

Sketch Two

This shows the smaller, inner grid, in (1/sq.root two) relationship, with Blackness Castle indicated at the north-west corner.



The length of side of this grid is where the Scot's measure system reveals the phi connection, discussed in previous post. Namely, (16* sq.root 3* 33) / (2* 37) equals 12.35842 miles(S), (half of which is 6.17921).
Incidentally, the discrepancy from phi exact is 6.5 feet, which is lost in the practical margins of error in my method. I work at all times with the limit in practice suggested as the optimum that medieval surveyors could achieve by known methods, by Professor Lind, in connection with the Bornholm work of Erling Haagensen and Henry Lincoln, in The Templar's Secret Island. This limit is 1 in 2000, or 99.95%, as discussed previously. 6.17921 mile(S) is 99.982% of 6.18034 miles(S).

The Scot's measure system I use is that defined by John Reid, 1683, in The Scot's Gard'ner, with the Scot's mile being in the ratio of 37 : 33 with the English/Imperial system. Inches and feet are common. Which raises many questions, not considered here!

Also, extreme accuracy that can be expected from O.S. maps is one metre, as explained to me by a professional cartographer friend, so any coordinates used here will be less than this. I would allow myself a discrepancy of up to ten metres. 6.5 feet is less than two metres (1.98 metres, to be precise)!

The issue of what accuracy the designers/surveyors worked to is not known, but the number of sites/points that are 'spot-on' indicates they got lucky very often.

I have been encouraged to allow for 'telluric'* off-set, and/or geographic/landscape practicalities in the past. I have allowed myself some leeway as at Blackness Castle(NT055 792), or Seafield Tower(NT280 885), where the exact geometric point is off the coast. I have also allowed the consideration of the 2.5 degree spread, and that these two points sit within this spread, as marker points. And, as at Arther's' Seat, the natural area of Holyrood Park, to be a 'unit-point-area', when considering such distances as the 200 mile plus spanning most of Scotland, and previously discussed.

* related to earth forces in some way! No further comment, for now!

These diagrams are simple representations of the geometry found so far.

Sketch Three

In late 1999 I moved to Selkirk to write up my findings till then. As previously mentioned I got connected to the Internet, made contact with William S. Buehler, and the geometry expanded, commensurate with my growing awareness of the landscape of the Tweed Valley and the countryside between Selkirk and Edinburgh. I was a member of the Sauniere Society at the time, and was privileged to hear Alistair Moffat discussing his book, Arthur And The Lost Kingdom, where my attention was drawn to Kelso, and the Roxburghe Castle area, east of Selkirk, where the Tweed and Teviot rivers meet.

On investigation I found that Kelso Abbey(NT727 339) was at the corner of the (2 by 1) grid point, south-east of St. Mary's Chapel:



A quick calculation here, to establish the accuracy of this. I am doing this from memory, and only using a list of O.S. coordinates, and re-doing the calculations as I go. And, I am aware that Kelso Abbey is not the exact point, as it is in the Schiehallion system described previously, but that a point closer to Roxburghe Castle(NT713 337) is the exact point. I shall first consider Kelso Abbey, to establish that we are in the right area:

3275.00 6570.19 St. Mary's Chapel
3728.87 6337.92 Kelso Abbey
--------- ----------
-453.87 232.27

Us usual using Pythagoras' Theorem, a grid unit distance of 509.85 hundreds of metres; which converts to 31.681 miles(E). Converting to Scots measure; using (33/37) gives 28.2557 miles(S).



What needs to be established is the approximation to the ( 2 by 1) diagonal which this distance represents, and the angle to O.S. grid:

We know that the side of the grid square is 12.38542 miles(S), (6.17921 * 2), and that the ( 2 by 1 ) diagonal is side times square root five, and the angle has a tangent of (1/2), or its complementary angle with a tangent of (2/1), which are 26.565 degrees, and 63.435 degrees respectively!

(6.17921 * 2) * square root five = 27.6343 miles(S). This is 0.6214 miles(S) short of Kelso Abbey!

The angle to O.S. grid has as tangent: (453.87/232.27) = 62.8987 degrees. or its complement to 90 degrees of 27.1013 degrees. This latter figure can be added to 90 to allow comparison with the north axis of orientation of the O.S. grid; 90 + 27.1013 = 117.1013. The exact angle for this vector should be (90 + 26.56505) 116.56505 degrees. The difference is 0.53625 degrees, well within the 2.5 degree spread discussed above!

Kelso Abbey could well be considered a marker point, for this grid!

I am content to leave this for now. Should anyone care to do some calculations for themselves I give a few more points around Roxburgh Castle, and also Floors Castle just to the north of Roxburgh Castle:

3716.82 6339.96 'hillock' east of Roxburgh Castle
3710.00 6335.22 'hillock' south of Roxburgh Castle
3713.04 6337.38 mean of two previous

3711.12 6346.62 Floors Castle

Sketch Four

In this part Ericstane Hill(NT059 122) shall be considered in relation to Kelso Abbey as marking the (3 by 1) diagonal south- west, and St. Mary's Chapel, as the (2 by 1) point south and west.

Ericstane Hill is north of Moffat between the A701, and the A74(M), with the 'Devil's Beef Tub' where stolen cattle were apparently penned in seclusion, in the valley to the north-east. It is the area where the Annan river rises, and close to the source of the Tweed. There is a Roman fortlet on its flank, with also the Eric Stane and monument.




3728.87 6337.92 Kelso Abbey
3059.81 6121.89 EricStane Hill(summit)
---------- ----------
669.06 216.03

By Pythagoras' theorem; 703.072 O.S.units(hundred metres); 43.68687 miles(E); 38.964 miles(S).

Now, the diagonal of a (3 by 1) rectangle is square root ten, so dividing we get 12.32132 miles(S), against the square unit side of 12.3584 miles(S), a difference of 0.03708 m(S), a discrepancy of 71.2 ells, or 73 yards, too short.

In real terms it is a discrepancy of 230 yards over the full distance, not great, not bad, good enough for immediate purposes.

The angle to O.S. grid is tangent (669.06/216.03), which gives an angle of 72.1055, and complement to 90 degrees of 17.8945.

The angle of the line from St. Mary's Chapel to Kelso Abbey, from above was found to be 27.1013 degrees.
Now, interestingly, the adjacent angles of a (2 by 1) and (3 by 1) is 45 degrees exactly; so 27.1013 plus 17.8945 equals 44.9958, 45 - 0.0042 degrees
As a check, Ericstane Hill can be compared to St. Mary's Chapel:

3275.00 6570.19 St. Mary's Chapel
3059.81 6121.89 EricStane Hill(summit)
---------- ----------
215.19 448.30

By Pythagoras' theorem 497.2722 O.S. grid units(hundred metres); or 30.899miles(E); or 27.5586 miles(S).

Dividing by square root five(the length of a (2 by 1) diagonal) gives 12.3246 miles(S), a discrepancy of 0.03381 miles(S) from grid square length, 12.3584 miles(S), some 65 ells, or 67 yards, short, similar in scale to the Kelso Abbey measure.

The angle to O.S grid is tangent (215.19/448.3) = 25.64163 degrees, which added to the St. Mary's Chapel - Kelso Abbey angle of 62.8987 degrees is 88.54 degrees, against the 90 degrees it should be. a shortfall of 1.46 degrees, well within the spread of 2.5 degrees, disussed above.

I could 'tweak' things a bit, and get the exact spots at both Kelso and Ericstane, and have done so previously, it's all in my notes somewhere, but for now I shall leave this, content in having established these two areas as containing the corners of the grid centred on St. Mary's Chapel discussed above.

Sketch Five

This sketch shows the next task, establishing the (3 by 1) axis through St. Mary's Chapel, from the mid-point of the Kelso - Ericstane line shown here:

St. Mary's Chapel, Mount Lothian grid contd.

So, having established That there is a grid in Lothian, based on the the exact same dimensions as that found at Bornholm, with St. Mary's Chapel and St. Baldred's Chapel on The Bass Rock forming the diagonal, which links with the side of a square through Dunsappie hill-fort and extends to Seafield Tower between Kinghorn and Kirkcaldy, I shall now show the other diagonal, north-west of St. Mary's Chapel. This diagonal can be fixed by Blackness Castle, and a natural sub-division is found, namely the side of the square fixed by Seafield Tower, becomes the diagonal of a smaller nested square, which shall then be used in the next quite astonishing development. For this exercise though, I shall use the North Berwick Law orientation, confirming that in the landscape, both are relevant. See below!

3275.00 6570.19 St. Mary's Chapel
3055.66 6792.48 Blackness Castle
---------- ----------
219.34 -222.29

Using Pythagoras' Theorem: 312.29 O.S grid units(100 metre), which converts to:
19.4046 miles(E), which is some 337 yards short of the exact figure of 19.596 miles(E)! Now as Blackness juts out into the Firth of Forth, the exact point is in fact off-shore, just like at Seafield Tower.



Through calculation the exact grid reference is found to be :

3275.00 6570.19 St. Mary's Chapel
3055.71 6796.88 Blackness(calculated point)
---------- ----------
319.29 -226.69

again, using Pythagoras' theorem: 315.40 grid units of 100 metres, which converts to: 19.598 miles(E), and allowing for the small rounding off in the calculations is good to 0.002 miles! (I have restricted the figures to two decimal places for convenience here, and to 3 decimal places in the final miles calculation! A discrepancy of some 10 feet. I trust this is acceptable! I normally work to 10 figures on the calculator!)

And the angle to grid north is 44.05 degrees west of grid north, which corresponds to ninety degrees difference to the North Berwick line, 45.95 degrees east of grid north.

The grid squares having diagonal St.Mary's Chapel to Blackness are shown below, with sides equal to 19.598 miles(E)/square root 2 = 13.858 miles(E):



The next section uses this smaller square as the grid unit for the next stage, which extends this grid in all directions.

Now, a final point for now, as I have just realized:

This grid square side length of 13.858 miles(E) is equal to 12.36 miles(S)(33/37 is the onversion factor, see explanation in previous posts, and why I here, always distinguish between the two systems by the (E) and (S).

Now, half of 12.36 miles(S) is 6.18, a harmonic of phi, or little phi, or 1/Phi!!!

This is a new finding, although I may have it in my notes, but I don't recall having found this previously in relation to this system, and has to be of significance, to my mind! Quite astonishing, but then again, that's nothing new in this whole research!

I shall work on this, and see what else is to be found!

Good grief, the time on my computer at this exact moment is 6.19am, BST!!!

I had just done some calculations, one of the dogs barked, and I checked the clock!!!!

This is what I found:

The Bornholm grid axis is 16*square root three miles(E). This equates to 24.71683315miles(S). This divided by 40 gives 0.617920828. The reciprocal is 1.6183303, which squared is 2.61899296. Now that is an approximation of Phisquared, or Phi^2. This multiplied by 6/5; or 1.2 is 3.142791552, which multiplied by 7 = 21.99954086, which is 99.998% of 22. 22/7 is a rough, and often used form of Pi.

So, this grid, and hence the Bornholm grid, in Scottish measure is based on a common form of Pi, and Phi! I had found some correlation with Scottish measure when I was working on the Bornholm grid, but nothing so convincing!

So the full factors involved must all resolve in some way:

[(16*sq.rt3*33/37*40)^2]*5/6 = 7/22; so; (16^2,*3,*33^2,*5,*22)/(37^2,*40^2,*7) = 1;

which resolves to (2^2,*3^2,*11^3)/(5,*37^2) = 47916/47915; which equals 1.00002087, the reciprocal of which is 0.99997913, equivalent to 99.9979% of 1.

Interesting exercise! Or perhaps I should get a life!?

Then again, astonishing find!

St.Mary's Chapel Bass Rock system further consideration

Having established the Bass Rock line, and considering this to be the diagonal of a square, the vertical will be 45 degrees anti-clockwise, a line which proves to run through the Arthurs' Seat area, and in fact a special rock on Dunsappie Fort, which I call pulpit Rock, whih intrigued me when first found back in the mid-nineties. The line extended north finishes just east of Seafield Tower, just offshore.





A lot of calculations were done using both the Bass Rock and North Berwick Law alignments, but the easiest way here is to just show the Dunsappie calculations first then the Seafield Tower point, just to keep things simple:

3275.00 6570.19 St. Mary's Chapel
3281.32 6731.72 Dunsappie 'notch' or Pulpit Rock
---------- ----------
-6.32 -161.53

Using Pythagoras again the distance is 161.6536, in One hundred metre units which converts to 10.0447 miles(E). This may be of interest but for now it is the angle this line makes to the O.S grid for comparison to the Bass Rock line:

6.32/161.53 = 0.039126, which is the tangent of 2.2406 degrees.

Comparing to the Bass Rock angle of 47.1803 - 2.2406 = 44.94 degrees, which is 0.06 degrees, or 1/100th of one clock-face-minute!

As the diagonal of a square is in square root two relationship with the side, this line extended north to a distance of 19.59592 miles(E), (16*sq.rt.3/sq.rt.2), the point indicated on the map just off-shore at Seafield Tower, which lies between Kinghorn and Kirkcaldy, is found.

Now that the St.Mary's -Bass Rock-Seafield Tower 45 degree right-angled triangle has been established, the full square can be projected, to complete the square on the circle of Bill Buehler's original circular system. And also, some of the sub-divisions also show points of interest.



click on image to see larger version!

This schematic was drawn prior to the calculations, and was what I needed to verify. It is good enough for now, as it shows the extended square and circle and some natural sub-divisions.

AS stand for Arthurs' Seat, and can be seen to be halfway between St. Mary's Chapel and Seafield Tower. It should be pointed out that the exact halfway point is some 440 yards south of Dunsappie, or one quarter mile, which is Duddingston loch, a beautiful spot famed in Scottish art for the painting by Raeburn of the Reverend Walker skating on Duddingston loch. And on the north-east shore lies Duddingston Kirk, the minister at the time was the Reverend John Thomson, who was also an amateur painter and had a studio down by the shore, still extant, an octagonal building which was also the home of the first curling club in the world.

Intriguingly, on the north wall of the kirk is a carved symbol, the same as is shown in 'The Templars' Secret Island', the book of the geometry of Bornholm, by Erling Haaagensen and Henry Lincoln, page 13, where there are examples of stones from Bodilsker, Nylars, Osterlars and Vestermarie, which 'echo the Cross of the Knights Templar'.





It should also be pointed out that there is also the Line from St. Mary's Chapel through Rosslyn Chapel and Arthurs' Seat summit which is the Roseline commonly or as Bill Buehler calls it the Tavhara Line, which passes just to the west of Seafield Tower, previously mentioned. This may constitute a more generous Selah Spoke, with the North Berwick Law line running between Arthurs' Seat and Dunsappie, and we can in passing check this:

3275.00 6570.19 St. Mary's Chapel
3275.28 6729.43 Arthurs' Seat summit
---------- ----------
-0.28 -159.24

and using Pythagoras' theorem: 159.24 O.S.units of 100 meters, which equates to 9.895 miles(E), and the angle to O.S. grid north being: 0.28/159.24 = 0.00176, which is the tangent of 0.1 degrees.

So the gap between this line and the Dunsappie line being 2.24 - 0.1 = 2.14 degrees, which may be considered as a 'Selah spoke'!

I consider the Arthurs' Seat area to be a 'unit point area' at large landscape scale.

So this gap being contained within the Arthurs' Seat area at a landscape scale this may be considered valid!


Also, and more pertinent to the next section is the Blackness Castle/Drem line. This can be seen to be between the diagonals at a distance the same as Seafield Tower, taken as radius. The squares on these sections of diagonals prove to be the unit squares of the next sub-system to be described! See next section!